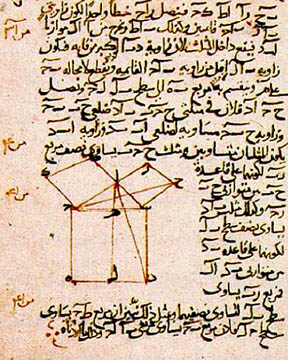
Euclid's proof of the Pythagorean theorem was rendered into Arabic in AD 1258 by the Persian mathematician al-Tusi.
“The word intuition, as used by mathematicians, carries a heavy load of mystery and ambiguity. Sometimes it seems to be a dangerous and illegitimate substitute for rigorous proof. In other contexts it seems to denote an inexplicable flash of insight by which the happy few gain mathematical knowledge which others only attain by long efforts. ”
CLASS ACTIVITY i: SOLVE A QUADRATIC
Students will begin by working individually on a timed mathematical task, reflecting on their thinking processes as they go. Later, they will find a partner and will work through a series of guiding questions. The questions are designed to generate some meta-thinking about approaches to the problem, rather than merely obtaining an answer.
The intention here, of course, is not to teach new mathematical facts. Rather like Figs viewed from multiple perspectives in Starting TOK, the point is to experience looking at something familiar—but not too familiar—in new ways!
1. Tell students to not write anything yet. Ask them to look at intensely, and think carefully about, how to approach solving this quadratic equation.
2. Allow students an accurately timed six minutes to solve the equation showing all stages of their working, using the step-by-step formal conventions used in their IB math classes. Announce that, if time permits, they should continue solving the same equation using multiple methods.
In the TOK class there will be a full spectrum of mathematical competence. The level of difficulty of this quadratic equation has been chosen so that some students may struggle and others may work through at least four methods.
3. After six minutes is up, students should tackle the questions in groups of four by combining pairs.
GENERATIVE QUESTIONS
If you were an octopus how would you have approached solving the quadratic?
If you were one of the Kindergarteners that you visited in the What do little kids know? unit in Starting TOK, how would you have approached solving the quadratic?
How did you approach solving the quadratic?
Try to list the many different ways you can use to solve quadratic equations. Don't worry if you did not get to them all.
GOING DEEPER
To what extent are the various elements of the quadratic equation an extension of the idea of counting numbers?
What is the relationship between algebra and simple arithmetic?
What is the relationship between algebra and geometry?
CLASS ACTIVITY II: SUM OF THE ANGLES IN A TRIANGLE
Bring to class paper, scissors and a few rulers to share.
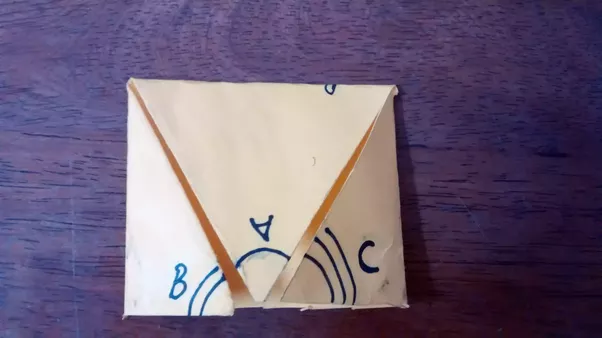
1. Again tell students not to write anything yet. Ask them to look at intensely, and think carefully about, for a timed 30 seconds, how to reverse engineer the paper model pictured above.
2. Tell students to grab a piece of paper quickly, and cut out a random triangle; then they should casually rip off the corners, and arrange the angles, more or less, in a straight line.
3. Next repeat the process in #2 much more systematically by following the four stage process in these four photographs.
Allow a full 10 minutes for the following tasks. Students are still working individually.
4. Next, return to the geometry you learned in school. Draw a simple geometric diagram with any construction lines you need to prove to your satisfaction visually that the sum of the angles of a triangle add up to 180 degrees.
5. Without using any mathematical symbols write out your proof in full English prose so that your reader would have to visualize your diagram and proof based on only the words you have written on the page (as if it had been written in a novel without the aid of a diagram).
Tell students to sit back-to-back with their partners. Here are the instructions:
You will read your prose proof from #5. The rule for this task is that your partner can ask you to pause or repeat what you read; but you cannot change what was written. Your partner must sketch or otherwise validate your proof only by listening to your text.
When finished, switch roles. Critique the validity of each other's work.
6. Finally, write out your proof using mathematical symbols as you would in a real math assignment. Use a refined version of your diagram, construction lines, letter symbols and only the barest minimum of elegant prose.
GENERATIVE QUESTIONS
Does folding down the corners of a single triangle or ripping off the corners and arranging them in a straight line count as proof that the sum of the angles of a triangle add up to 180 degrees?
Is performing the corner ripping technique more convincing as proof when it is done carefully and accurately, using the ruler to construct the triangle by joining the randomly points marked by crosses?
Would ripping off the corners of hundreds of random single triangles and arranging them in a straight lines count as proof that the sum of the angles of a triangle add up to 180 degrees?
A straight line is half a turn in this context; but where does the precise designation of 180 degrees come from?
In terms of rigor and validity, what are the essential differences between the proof techniques you used in #5 and #6?
What do we mean by elegance in proof?
Printable pdf of instructions for the activities all the generative questions.
CLASS ACTIVITY III: THE MONTY HALL PROBLEM
Students may have previously encountered the Monty Hall Problem in The Curious Incident of the Dog in the Night-Time (2003). Mark Haddon's protagonist has Asperger's syndrome and is gifted mathematically. The novel contains some memorable passages outlining the Monty Hall Problem in the hero's voice. (Task #5 in the second class activity, requiring a math proof in prose, was inspired by this.)
A version of the Monty Hall problem was a regular feature of the American television game show Let's Make a Deal, named after its host, Monty Hall. It was later published in Marilyn Vos Savant's column in Sunday Parade.
“Behind one door is a car; behind the others, goats. You pick a door, say No. 1, and the host, who knows what’s behind the other doors, opens another door, say No. 3, which has a goat. He then says to you, ‘Do you want to pick door No. 2?’ Is it to your advantage to take the switch?”
Thousands of letters expressing outrage and disagreement were sent to Marylin Vos Savant after she published her solution to the Monty Hall Problem. Many came from tenured academics.
“You blew it, and you blew it big! Since you seem to have difficulty grasping the basic principle at work here, I’ll explain. After the host reveals a goat, you now have a one-in-two chance of being correct. Whether you change your selection or not, the odds are the same. There is enough mathematical illiteracy in this country, and we don’t need the world’s highest IQ propagating more. Shame!”
Students should view the video. They should pause at exactly 1: 20 so they can decide what they would do.
GENERATIVE QUESTIONS
To what extent does the explanation for the Monty Hall Problem, outlined in the video, hold up as proof?
To what extent are probability and certainty in the statistical branch of mathematics mutually exclusive?
THINKING ABOUT PROOF AND INTUITION
We can think of the term ‘intuition’ as a catch-all label for a variety of effortless, inescapable, self-evident perceptions or insights that seem to arrive all at once and fully-formed. The origins of most of our intuitions are hidden below the threshold of conscious awareness, but remain a vivid aspect of our subjective experience.
The element of intuition in proof partially unsettles notions of consistency and certainty in mathematics. Reason is supposed to privilege rigor and objectivity and prefers to subjugate emotions and subjective feelings.
In Euclid's Geometry the original axioms/postulates—the foundations for the entire edifice—are viewed as commonsensical or self-evident. There are certain pivotal junctures in routine geometrical proofs that depend on intuitive leaps. The Reuben and Hersh quote should resonate strongly with students after completing Task #6 in sum of the angles in a triangle.
“The extraneous lines which in high school are often called “construction lines” complicate the figure, but form an essential part of the deductive process… Now, how does one know where to draw these lines so as to reason with them? It would seem that these lines are accidental or fortuitous. In a sense this is true and constitutes the genius or trick of the thing. Finding the lines is part of finding a proof, and this may be no easy matter. With experience come insight and skill at finding proper construction lines. ”